Centripetal acceleration and centripetal force

Every time an object moves along a non-linear trajectory, we are sure some force acts on the object. This happens even if the speed of the object is constant: the object must experiences some forces because its direction of motion changes!

Every time an object describes a curved trajectory it undergoes an acceleration. In effect along the curve its direction of motion changes, that means a change in velocity, that means an acceleration.

What are direction and magnitude of this acceleration? We will try to answer the question in the simple case of uniform circular motion.

Uniform circular motion and centripetal acceleration

An object describing a circumference with a constant speed represents this kind of motion.

For such an object the direction of the velocity vector continually changes, so the object continually experiences an acceleration.

Direction of this acceleration:

since the definition of instantaneous acceleration is

the direction of is the same of change in velocity vector .

The direction of the can be computed as follows:

We chose a very short time interval between two positions.

We compute the difference between the velocities and at those positions (see the figure below).

The direction of the acceleration . is equal to the direction of the change in velocity .

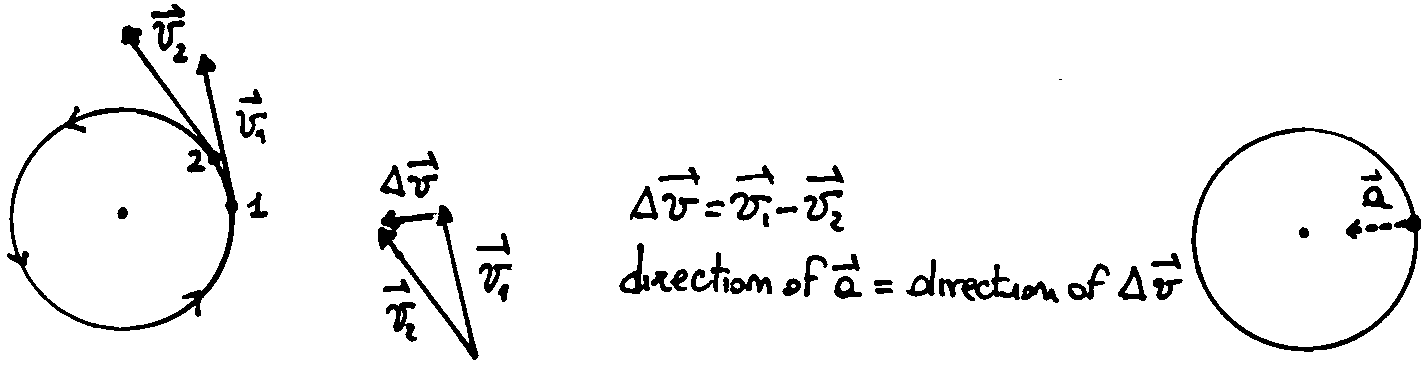
(best draws are available on page 76 in the textbook).

Following this line of reasoning we conclude that the acceleration is always directed toward the centre of the circle and for this reason we call this acceleration CENTRIPETAL ACCELERATION .

Magnitude of the centripetal acceleration .

The centripetal acceleration has

1. an inverse dependence on the radius of curve: To produce a tight curve (small radius) we need a large centripetal acceleration and vice versa.
2. A direct dependence on the square of the speed. In effect an increasing speed modify the vector in two different ways at the same time. Looking at the triangle of the velocities (figure below) we can say that a larger speed implies that and becomes longer hence is longer too (fig B); furthermore a larger speed implies a larger angle between the two vectors and hence becomes still longer (fig C).

Therefore the magnitude of the centripetal acceleration has a double and direct dependence on speed and an inverse dependence on the radius. Using maths:

.

Centripetal force.

According to the Newton’s Second Law, if an mass object covers a curved trajectory, a centripetal force

is acting.

1. Direction of the centripetal force:  the same of centripetal acceleration, toward the centre of the curve.
2. Magnitude of the force:

where is the speed of the object, and is the bend radius of the covered curve.